Simplify Square Root Of 169

In mathematics, the square root of a number is a value that gives the original number on multiplication by itself. If p is the square root of q, then information technology is represented as p = √q, or we tin can express the same equation every bit p 2 = q. Here,'√' is the radical symbol used to denote the root of numbers. The positive number, when multiplied by itself, represents the square of the number . Thus, the foursquare root of the square of a positive number gives the original number. In this article, yous will learn how to summate the square root of 169 in unlike methods and the solved examples on the foursquare root of 169.

What is the Foursquare root of 169?

The square root of 169 is 13, i.due east. √169 = 13. The radical representation of the square root of 169 is √169. Also, we know that the foursquare of thirteen is 169, i.e. 13 2 = thirteen × xiii = 169. Thus, the square root of 169 can besides be expressed as, √169 = √(13) two = √(13 × 13) = xiii. Also, we can say that 169 is a perfect square . However, the square root of 169 is ±13 . Every bit we know, 13 × 13 = 169 and (-13) × (-xiii) = 169. From this representation, we can say that the square root of 169 can exist both positive and negative of xiii. In general, "the foursquare root" is often used to refer to the principal or not-negative square root. Hence, nosotros write the positive number equally the result unless it is specified as roots.

How to Summate Square root of 169

We can summate the square of 169 in different ways such as the subtraction method, prime number factorization and long division method. All these methods are explained here with detailed explanations.

Square root of 169 by Repeated Subtraction Method

In this method, we should subtract the successive odd numbers till we obtain nil, starting from 169 and one. The number of odd numbers we subtract in this process is treated as the square root of 169.

169 – 1 = 168
168 – 3 = 165
165 – v = 160
160 – 7 = 153
153 – 9 = 144
144 – 11 = 133
133 – thirteen = 120
120 – fifteen = 105
105 – 17 = 88
88 – nineteen = 69
69 – 21 = 48
48 – 23 = 25
25 – 25 = 0

Thus, starting from 169, we have subtracted 13 times to obtain 0. Therefore, the foursquare root of 169 is thirteen.
Click hither to become more data about the square root of a number by repeated subtraction method.

Square root of 169 by Prime factorization

In the prime number factorization method, we have to divide the number by prime numbers starting from 2. When it cannot be divided further with 2, separate it with the next prime number, i.e. iii and continue this process till we get 1.
169 ÷ 2 = 84.five
169 ÷ three = 56.33
169 ÷ v = 33.8
169 ÷ 7 = 24.1428
169 ÷ xi = fifteen.3636
169 ÷ 13 = 13
Here, xiii is the prime number and is ane of the prime factors of 169.
So, xiii ÷ 13 = one
Therefore, the prime factorization of 169 is 13 × 13.
That ways, 169 = 13 × 13
At present, take the square root on both sides of the above representation.
√169 = √(13 × 13) = 13.
Hence, the square root of 169 is equal to 13.

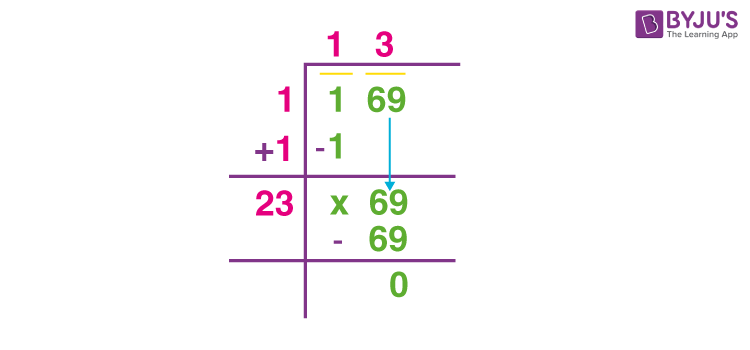
Square root of 169 by Long segmentation method

Get through the process given beneath, to learn how to notice the square root of 169 by the long division method.

Write 169.
Take the digits of the number in pairs from the right. Then, from 169, 69 is called every bit a pair, and i stands alone.
Nosotros need to separate 1 with a number such that the number × number gives 1 or a number less than that.
At present, we got ane as the quotient and 0 as the residue.
Let's bring down 69 for division.
Double the divisor, that means one + one = 2. Write this as one of the digits for the new divisor.
Find a number that tin can be placed adjacent right of 2 to obtain a two-digit number equally a new divisor. Too, the number set here should be multiplied by itself to produce 69 or fewer than that.
We find that 3 is the number such that 23 × 3 gives 69.
At present we got the quotient 13, and the remainder as 0.

Square root of 169

Get Square Root of More Numbers Here

Video Lessons on Square Roots

Visualising square roots

Finding Square roots

Solved Examples

Case 1:
The surface area of a foursquare is 169 square units. Find the length of the side of that square.
Solution:
Given,
Area of a square = 169 square units
Nosotros know that the area of a foursquare of side "a" = a two
So, a 2 = 169
a = √169 = 13 {a cannot be -13 since the side measure cannot exist negative}
Therefore, the side of the square is xiii units.

Instance 2:
Simplify: 14 + √169 – 7 × 3.
Solution:
14 + √169 – 7 × 3
= 14 + thirteen – 7 × iii
= 14 + 13 – 21
= 27 – 21
= half dozen
Therefore, 14 + √169 – 7 × 3 = half-dozen.
If we have √169 = -13, then:
14 + √169 – 7 × 3
= fourteen – xiii – vii × 3
= xiv – xiii – 21
= -20

Frequently Asked Questions on Foursquare root of 169 – FAQs

How do y'all discover the foursquare root of 169?

We can find the square root of 169 in 3 different ways, namely repeated subtraction, prime number factorisation and long division method.

What squared gives yous 169?

Squaring 13 gives us the issue 169, since the square root of 169 is 13. Hence, the square of 13 will exist 169. That means, thirteen^2 = thirteen × xiii = 169.

Is the cube root of 169 rational or irrational?

The cube root of 169 is irrational since it is not a perfect cube number.

Is 169 a perfect foursquare?

Yes, 169 is a perfect foursquare since the square root of 169 is xiii, which is a whole number.

Is the foursquare root of 169 a rational number?

Yes, the square root of 169 is a rational number. Every bit we know that √169 = 13, a rational number.